marek3.png copyright 07/06/2002 Wendy Krieger

In order to expose Marek's snub process more clearly, I created a series of snubs, based on triangles and squares, with the hexagons being the snub faces.

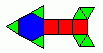
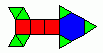
Figure A shows the normal extended snub. The order of faces around a hexagonal face goes 3-4-6-3-4-6, where--in this case--opposite sides of the hexagon have the same face.

In a Marek-snub, this happens only for one of the cases. Figures B, C, and D show the Marek-snubs where the order of the faces are 4-3-4-6-3-6, 3-4-3-6-4-6, and 3-6-3-4-6-4 respectively. One can see that the polygon that is opposite another of the same type. must be even. The case 4-3-4-6-3-6 fails, as the hexagon to the right of the big triangle should have either two hexagons or two squares on the edges near the triangle: it has one of each.

In practice, one could use ever larger polygons--octagons for example--in a pattern such as "a,b,c,d,a,d,c,b,a" against "a,b,c,d,a,b,c,d".

The mind boggles.

back to Gallery index
back to Tyler Applet
back to Geometry index
back to Superliminal home