It had been believed for centuries that all simple (I.E. non self intersecting) closed polyhedra composed of rigid faces and hinged edges are themselves rigid. This was known as the "Rigidity Conjecture". Then in 1977, Robert Connelly found the first counterexample. Soon afterwards, Klaus Steffen found an elegant flexible polyhedron with only nine vertices. I have not heard of any additional flexible models which are not variations of one of these two models.

There are two ways you can interact with the Steffen model on this site. The first is a Java applet which displays a 3D wireframe Steffen model and lets you pull and stretch the vertices around while it attempts to keep all its edges their proper lengths. Note that to view this applet you will need a browser that supports Java version 1.1 such as Netscape 4.5 or similar Microsoft Internet Explorer.

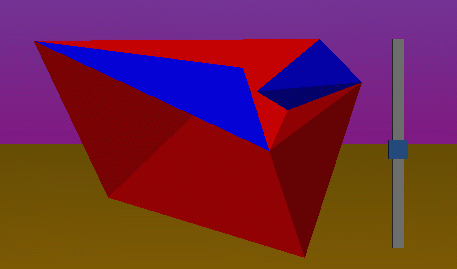
The second way, shown above, is a 3D VRML shaded version of the Steffen model I created using keyframe data generated using the above applet. You can move a slider which will cause the model to flex from one end of an animation sequence to the other. You can also click and drag on the model to rotate it around so that you can watch the animation from any angle. Notice that the outer surface of the model is colored red and the inner surface blue. Initially the model appears completely red which shows that the model is not intersecting itself. After flexing a bit you can begin to see parts of the model poke through itself showing some of its blue inner surface. To view this model you will need a VRML 2.0 browser plug-in such as the SGI Cosmo plug-in.