The 554 Problem

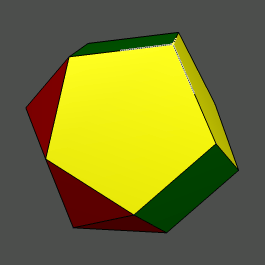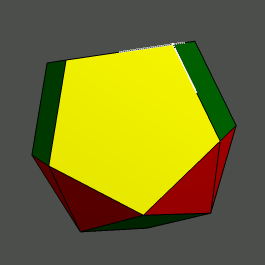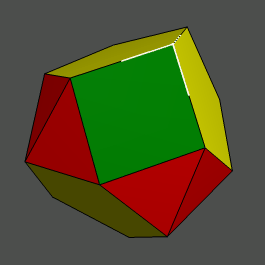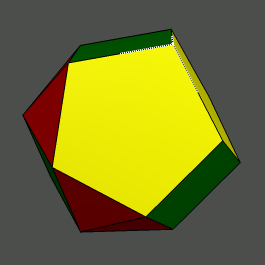
I have a nice collection of Polydron Polydron tiles and once wondered whether one could construct a polyhedron that contained at least one vertex surrounded by exactly two pentagons and a square which I called a 554. The faces can be any regular polygons, and can even be nonconvex and self-intersecting. Sounds easy, right? Well I tried hard to find an example and failed. Then in the 1980s I was on a polyhedron mailing list for a time run by Magnus Wenninger, and included John Conway, George Hart, Norman Johnson, and other geometry luminaries which I eventually got interested in the question. There was a flurry of activity for a time looking for solutions to all such polyhedra which Conway called "Acrohedra". I was a little miffed that he insisted in naming my invention, but I guess the name stuck. That activity resulted in solutions to many such acrohedra but never the 554. This was funny to me because for a long time Conway insisted that he could easily settle the question if he ever put his mind to the task, but once he did, he failed, as did everyone else. This suggests it might be impossible, though I don't see a reason for that either, and it's remained a mystery ever since. We did find many interesting near misses such as one of mine above, animated by Eduard Baumann. The faces appear to be regular polygons but are not quite flat. The model is spinning around a 554 vertex at the top, and highlighted by white edges.

I have now decided to offer a $200 prize for the first true 554 or a proof that it's impossible. If you find a solution, just email me here to claim your prize. You may also send questions or comments. Good luck!